3 队列
概述
计算机科学中,queue 是以顺序的方式维护的一组数据集合,在一端添加数据,从另一端移除数据。习惯来说,添加的一端称为尾,移除的一端称为头,就如同生活中的排队买商品
In computer science, a queue is a collection of entities that are maintained in a sequence and can be modified by the addition of entities at one end of the sequence and the removal of entities from the other end of the sequence
先定义一个简化的队列接口
public interface Queue<E> {
/**
* 向队列尾插入值
* @param value 待插入值
* @return 插入成功返回 true, 插入失败返回 false
*/
boolean offer(E value);
/**
* 从对列头获取值, 并移除
* @return 如果队列非空返回对头值, 否则返回 null
*/
E poll();
/**
* 从对列头获取值, 不移除
* @return 如果队列非空返回对头值, 否则返回 null
*/
E peek();
/**
* 检查队列是否为空
* @return 空返回 true, 否则返回 false
*/
boolean isEmpty();
/**
* 检查队列是否已满
* @return 满返回 true, 否则返回 false
*/
boolean isFull();
}
链表实现
下面以单向环形带哨兵链表方式来实现队列
代码
public class LinkedListQueue<E>
implements Queue<E>, Iterable<E> {
private static class Node<E> {
E value;
Node<E> next;
public Node(E value, Node<E> next) {
this.value = value;
this.next = next;
}
}
private Node<E> head = new Node<>(null, null);
private Node<E> tail = head;
private int size = 0;
private int capacity = Integer.MAX_VALUE;
{
tail.next = head;
}
public LinkedListQueue() {
}
public LinkedListQueue(int capacity) {
this.capacity = capacity;
}
@Override
public boolean offer(E value) {
if (isFull()) {
return false;
}
Node<E> added = new Node<>(value, head);
tail.next = added;
tail = added;
size++;
return true;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
Node<E> first = head.next;
head.next = first.next;
if (first == tail) {
tail = head;
}
size--;
return first.value;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return head.next.value;
}
@Override
public boolean isEmpty() {
return head == tail;
}
@Override
public boolean isFull() {
return size == capacity;
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
Node<E> p = head.next;
@Override
public boolean hasNext() {
return p != head;
}
@Override
public E next() {
E value = p.value;
p = p.next;
return value;
}
};
}
}
环形数组实现
好处
- 对比普通数组,起点和终点更为自由,不用考虑数据移动
- “环”意味着不会存在【越界】问题
- 数组性能更佳
- 环形数组比较适合实现有界队列、RingBuffer �等
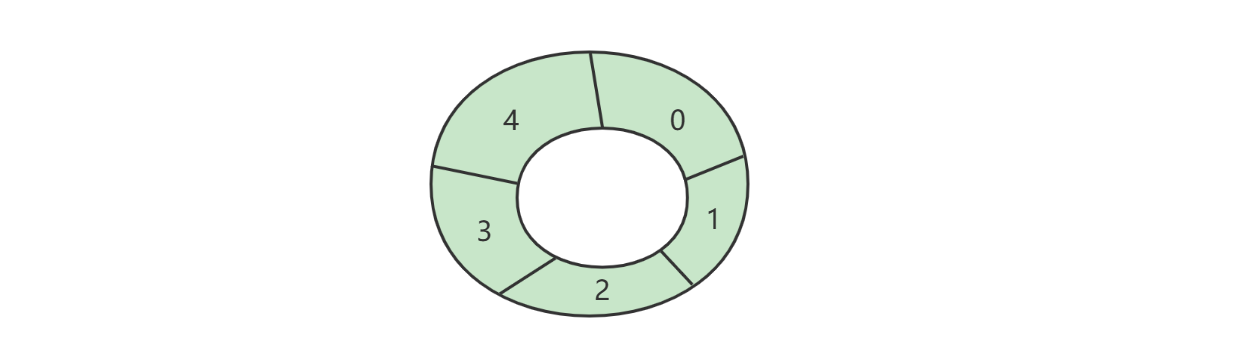
下标计算
例如,数组长度是 5,当前位置是 3 ,向前走 2 步,此时下标为
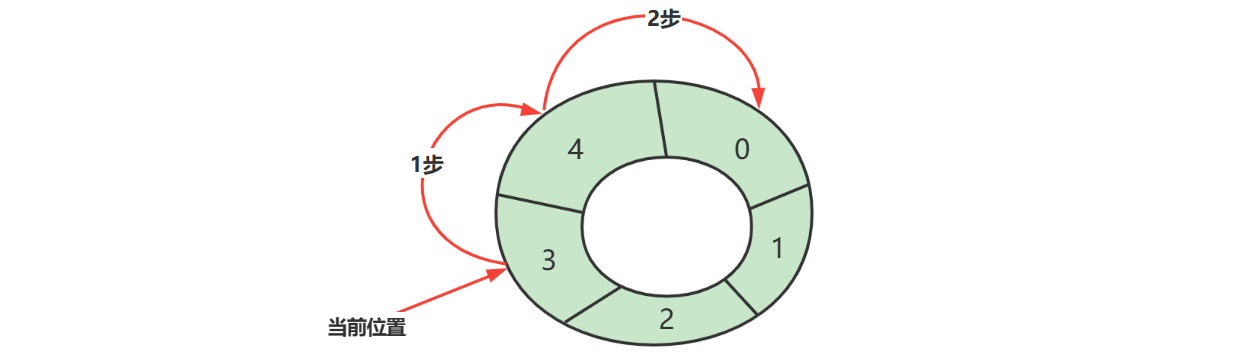
- cur 当前指针位置
- step 前进步数
- length 数组长度
注意:
- 如果 step = 1,也就是一次走一步,可以在 >= length 时重置为 0 即可
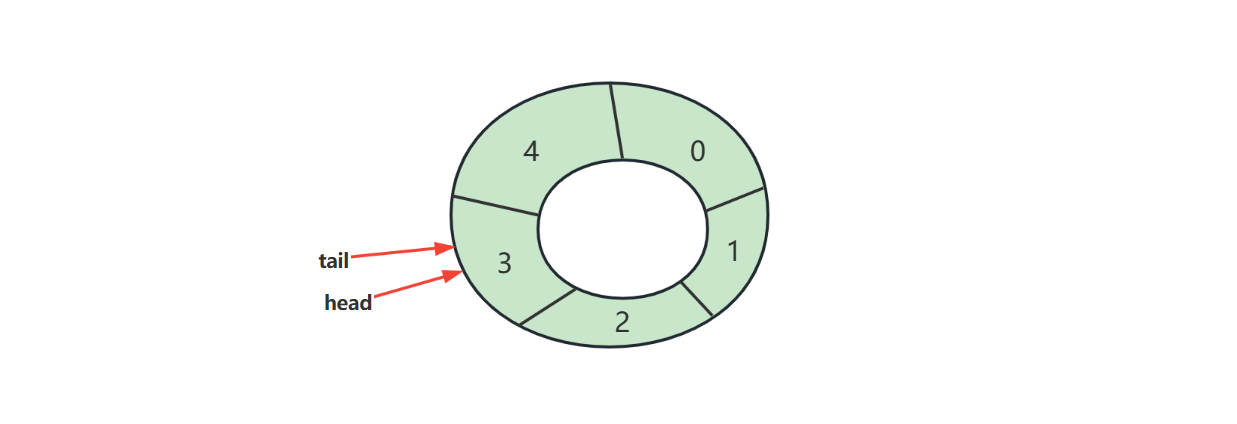
判断空
判断满
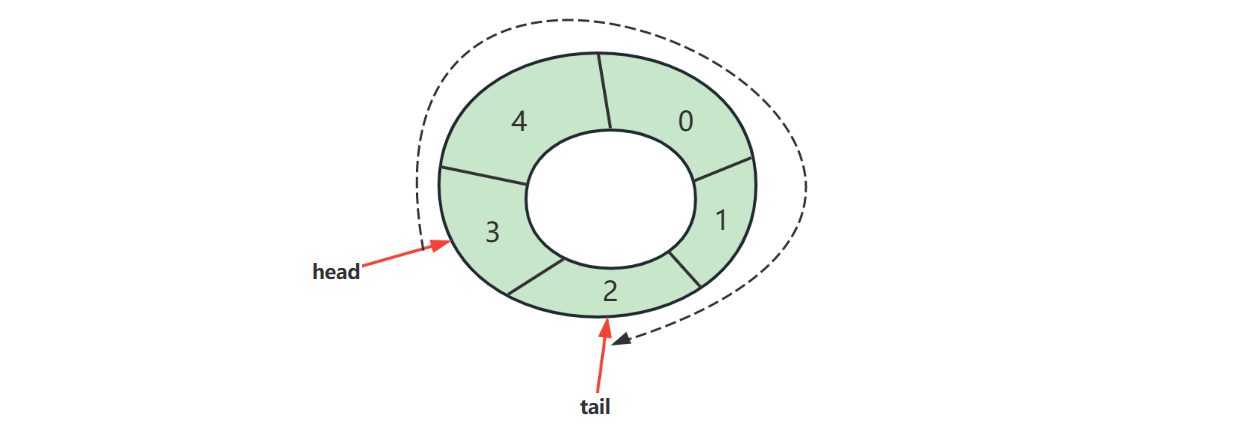
满之后的策略可以根据业务需求决定
- 例如我们要实现的环形队列,满之后就拒绝入队
代码
public class ArrayQueue<E> implements Queue<E>, Iterable<E>{
private int head = 0;
private int tail = 0;
private final E[] array;
private final int length;
@SuppressWarnings("all")
public ArrayQueue(int capacity) {
length = capacity + 1;
array = (E[]) new Object[length];
}
@Override
public boolean offer(E value) {
if (isFull()) {
return false;
}
array[tail] = value;
tail = (tail + 1) % length;
return true;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
E value = array[head];
head = (head + 1) % length;
return value;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return array[head];
}
@Override
public boolean isEmpty() {
return tail == head;
}
@Override
public boolean isFull() {
return (tail + 1) % length == head;
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
int p = head;
@Override
public boolean hasNext() {
return p != tail;
}
@Override
public E next() {
E value = array[p];
p = (p + 1) % array.length;
return value;
}
};
}
}
判断空、满方法2
引入 size
public class ArrayQueue2<E> implements Queue<E>, Iterable<E> {
private int head = 0;
private int tail = 0;
private final E[] array;
private final int capacity;
private int size = 0;
@SuppressWarnings("all")
public ArrayQueue2(int capacity) {
this.capacity = capacity;
array = (E[]) new Object[capacity];
}
@Override
public boolean offer(E value) {
if (isFull()) {
return false;
}
array[tail] = value;
tail = (tail + 1) % capacity;
size++;
return true;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
E value = array[head];
head = (head + 1) % capacity;
size--;
return value;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return array[head];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == capacity;
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
int p = head;
@Override
public boolean hasNext() {
return p != tail;
}
@Override
public E next() {
E value = array[p];
p = (p + 1) % capacity;
return value;
}
};
}
}
判断空、满方法3
-
head 和 tail 不断递增,用到索引时,再用它们进行计算,两个问题
-
如何保证 head 和 tail 自增超过正整数最大值的正确性
-
��如何让取模运算性能更高
-
-
答案:让 capacity 为 2 的幂
public class ArrayQueue3<E> implements Queue<E>, Iterable<E> {
private int head = 0;
private int tail = 0;
private final E[] array;
private final int capacity;
@SuppressWarnings("all")
public ArrayQueue3(int capacity) {
if ((capacity & capacity - 1) != 0) {
throw new IllegalArgumentException("capacity 必须为 2 的幂");
}
this.capacity = capacity;
array = (E[]) new Object[this.capacity];
}
@Override
public boolean offer(E value) {
if (isFull()) {
return false;
}
array[tail & capacity - 1] = value;
tail++;
return true;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
E value = array[head & capacity - 1];
head++;
return value;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return array[head & capacity - 1];
}
@Override
public boolean isEmpty() {
return tail - head == 0;
}
@Override
public boolean isFull() {
return tail - head == capacity;
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
int p = head;
@Override
public boolean hasNext() {
return p != tail;
}
@Override
public E next() {
E value = array[p & capacity - 1];
p++;
return value;
}
};
}
}
3.1 双端队列
概述
双端队列、队列、栈对比
| 定义 | 特点 | |
|---|---|---|
| 队列 | 一端删除(头)另一端添加(尾) | First In First Out |
| 栈 | 一端删除和添加(顶) | Last In First Out |
| 双端队列 | 两端都可以删除、添加 | |
| 优先级队列 | 优先级高者先出队 | |
| 延时队列 | 根据延时时间确定优先级 | |
| 并发�非阻塞队列 | 队列空或满时不阻塞 | |
| 并发阻塞队列 | 队列空时删除阻塞、队列满时添加阻塞 |
注1:
- Java 中 LinkedList 即为典型双端队列实现,不过它同时实现了 Queue 接口,也提供了栈的 push pop 等方法
注2:
不同语言,操作双端队列的方法命名有所不同,参见下表
操作 Java JavaScript C++ leetCode 641 尾部插入 offerLast push push_back insertLast 头部插入 offerFirst unshift push_front insertFront 尾部移除 pollLast pop pop_back deleteLast 头部移除 pollFirst shift pop_front deleteFront 尾部获取 peekLast at(-1) back getRear 头部获取 peekFirst at(0) front getFront 吐槽一下 leetCode 命名比较 low
常见的单词还有 enqueue 入队、dequeue 出队
接口定义
public interface Deque<E> {
boolean offerFirst(E e);
boolean offerLast(E e);
E pollFirst();
E pollLast();
E peekFirst();
E peekLast();
boolean isEmpty();
boolean isFull();
}
链表实现
/**
* 基于环形链表的双端队列
* @param <E> 元素类型
*/
public class LinkedListDeque<E> implements Deque<E>, Iterable<E> {
@Override
public boolean offerFirst(E e) {
if (isFull()) {
return false;
}
size++;
Node<E> a = sentinel;
Node<E> b = sentinel.next;
Node<E> offered = new Node<>(a, e, b);
a.next = offered;
b.prev = offered;
return true;
}
@Override
public boolean offerLast(E e) {
if (isFull()) {
return false;
}
size++;
Node<E> a = sentinel.prev;
Node<E> b = sentinel;
Node<E> offered = new Node<>(a, e, b);
a.next = offered;
b.prev = offered;
return true;
}
@Override
public E pollFirst() {
if (isEmpty()) {
return null;
}
Node<E> a = sentinel;
Node<E> polled = sentinel.next;
Node<E> b = polled.next;
a.next = b;
b.prev = a;
size--;
return polled.value;
}
@Override
public E pollLast() {
if (isEmpty()) {
return null;
}
Node<E> polled = sentinel.prev;
Node<E> a = polled.prev;
Node<E> b = sentinel;
a.next = b;
b.prev = a;
size--;
return polled.value;
}
@Override
public E peekFirst() {
if (isEmpty()) {
return null;
}
return sentinel.next.value;
}
@Override
public E peekLast() {
if (isEmpty()) {
return null;
}
return sentinel.prev.value;
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == capacity;
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
Node<E> p = sentinel.next;
@Override
public boolean hasNext() {
return p != sentinel;
}
@Override
public E next() {
E value = p.value;
p = p.next;
return value;
}
};
}
static class Node<E> {
Node<E> prev;
E value;
Node<E> next;
public Node(Node<E> prev, E value, Node<E> next) {
this.prev = prev;
this.value = value;
this.next = next;
}
}
Node<E> sentinel = new Node<>(null, null, null);
int capacity;
int size;
public LinkedListDeque(int capacity) {
sentinel.next = sentinel;
sentinel.prev = sentinel;
this.capacity = capacity;
}
}
数组实现
/**
* 基于循环数组实现, 特点
* <ul>
* <li>tail 停下来的位置不存储, 会浪费一个位置</li>
* </ul>
* @param <E>
*/
public class ArrayDeque1<E> implements Deque<E>, Iterable<E> {
/*
h
t
0 1 2 3
b a
*/
@Override
public boolean offerFirst(E e) {
if (isFull()) {
return false;
}
head = dec(head, array.length);
array[head] = e;
return true;
}
@Override
public boolean offerLast(E e) {
if (isFull()) {
return false;
}
array[tail] = e;
tail = inc(tail, array.length);
return true;
}
@Override
public E pollFirst() {
if (isEmpty()) {
return null;
}
E e = array[head];
array[head] = null;
head = inc(head, array.length);
return e;
}
@Override
public E pollLast() {
if (isEmpty()) {
return null;
}
tail = dec(tail, array.length);
E e = array[tail];
array[tail] = null;
return e;
}
@Override
public E peekFirst() {
if (isEmpty()) {
return null;
}
return array[head];
}
@Override
public E peekLast() {
if (isEmpty()) {
return null;
}
return array[dec(tail, array.length)];
}
@Override
public boolean isEmpty() {
return head == tail;
}
@Override
public boolean isFull() {
if (tail > head) {
return tail - head == array.length - 1;
} else if (tail < head) {
return head - tail == 1;
} else {
return false;
}
}
@Override
public Iterator<E> iterator() {
return new Iterator<E>() {
int p = head;
@Override
public boolean hasNext() {
return p != tail;
}
@Override
public E next() {
E e = array[p];
p = inc(p, array.length);
return e;
}
};
}
E[] array;
int head;
int tail;
@SuppressWarnings("unchecked")
public ArrayDeque1(int capacity) {
array = (E[]) new Object[capacity + 1];
}
static int inc(int i, int length) {
if (i + 1 >= length) {
return 0;
}
return i + 1;
}
static int dec(int i, int length) {
if (i - 1 < 0) {
return length - 1;
}
return i - 1;
}
}
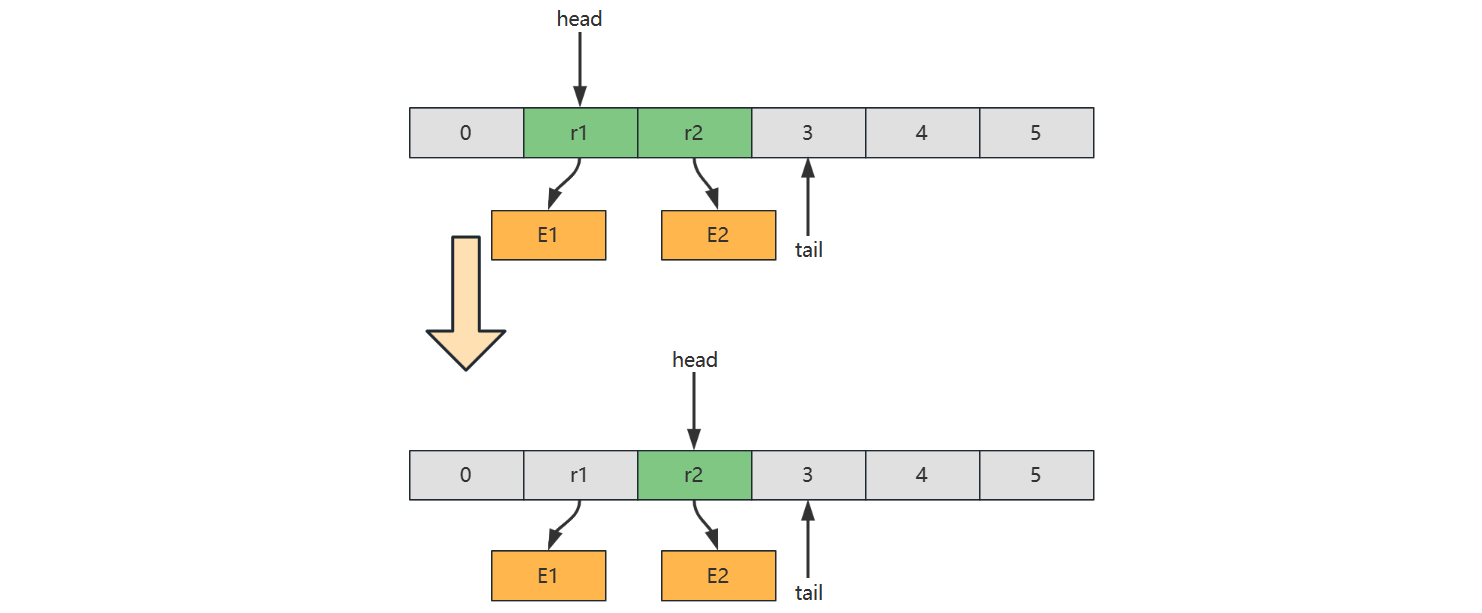
数组实现中,如果存储的是基本类型,那么无需考虑内存释放,例如
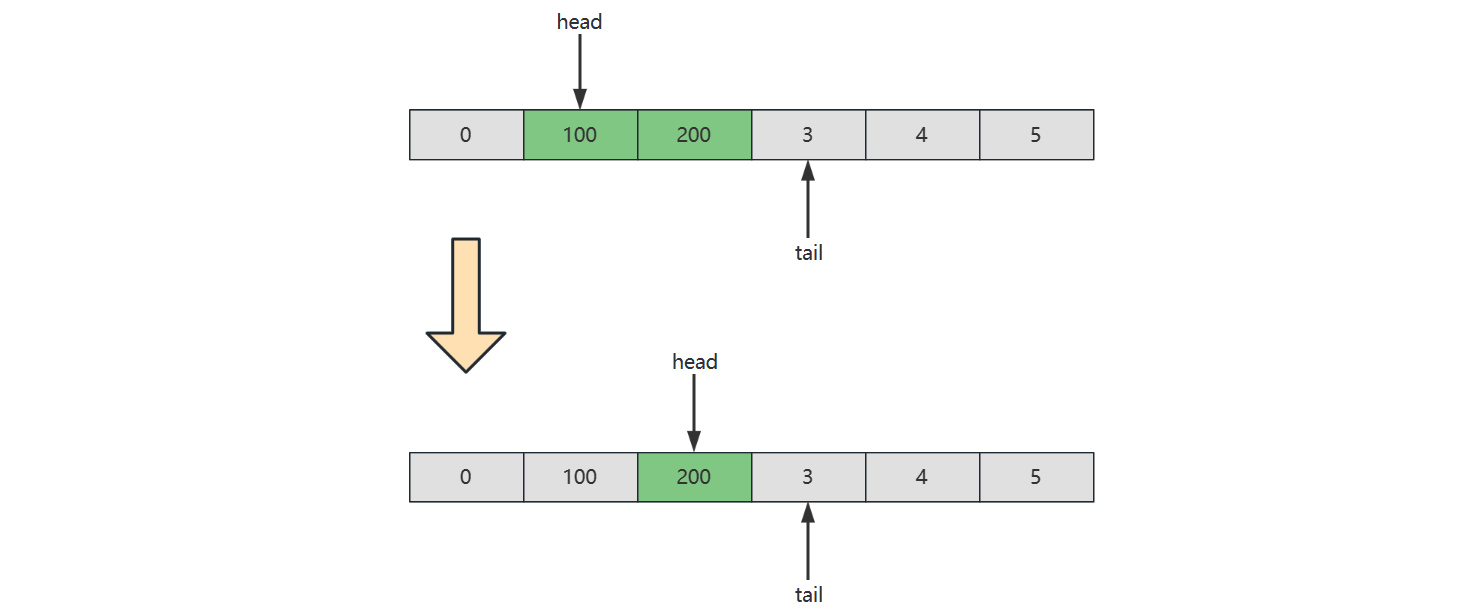
但如果存储的是引用类型,应当设置该位置的引用为 null,以便内存及时��释放
3.2 优先级队列
无序数组实现
要点
- 入队保持顺序
- 出队前找到优先级最高的出队,相当于一次选择排序
public class PriorityQueue1<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue1(int capacity) {
array = new Priority[capacity];
}
@Override // O(1)
public boolean offer(E e) {
if (isFull()) {
return false;
}
array[size++] = e;
return true;
}
// 返回优先级最高的索引值
private int selectMax() {
int max = 0;
for (int i = 1; i < size; i++) {
if (array[i].priority() > array[max].priority()) {
max = i;
}
}
return max;
}
@Override // O(n)
public E poll() {
if (isEmpty()) {
return null;
}
int max = selectMax();
E e = (E) array[max];
remove(max);
return e;
}
private void remove(int index) {
if (index < size - 1) {
System.arraycopy(array, index + 1,
array, index, size - 1 - index);
}
array[--size] = null; // help GC
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
int max = selectMax();
return (E) array[max];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
- 视频中忘记了 help GC,注意一下
有序数组实现
要点
- 入队后排好序,优先级最高的排列在尾部
- 出队只需删除尾部元素即可
public class PriorityQueue2<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue2(int capacity) {
array = new Priority[capacity];
}
// O(n)
@Override
public boolean offer(E e) {
if (isFull()) {
return false;
}
insert(e);
size++;
return true;
}
// 一轮插入排序
private void insert(E e) {
int i = size - 1;
while (i >= 0 && array[i].priority() > e.priority()) {
array[i + 1] = array[i];
i--;
}
array[i + 1] = e;
}
// O(1)
@Override
public E poll() {
if (isEmpty()) {
return null;
}
E e = (E) array[size - 1];
array[--size] = null; // help GC
return e;
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return (E) array[size - 1];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
堆实现
计算机科学中,堆是一种基于树的数据结构,通常用完全二叉树实现。堆的特性如下
- 在大顶堆中,任意节点 C 与它的父节点 P 符合
- 而小顶堆中,任意节点 C 与它的父节点 P 符合
- 最顶层的节点(没有父亲)称之为 root 根节点
In computer science, a heap is a specialized tree-based data structure which is essentially an almost complete tree that satisfies the heap property: in a max heap, for any given node C, if P is a parent node of C, then the key (the value) of P is greater than or equal to the key of C. In a min heap, the key of P is less than or equal to the key of C. The node at the "top" of the heap (with no parents) is called the root node
例1 - 满二叉树(Full Binary Tree)特点:每一层都是填满的
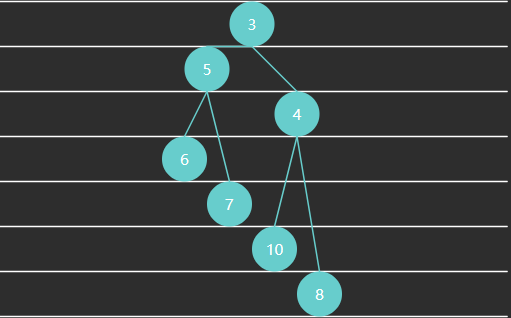
例2 - 完全二叉树(Complete Binary Tree)特点:最后一层可能未填满,靠左对齐
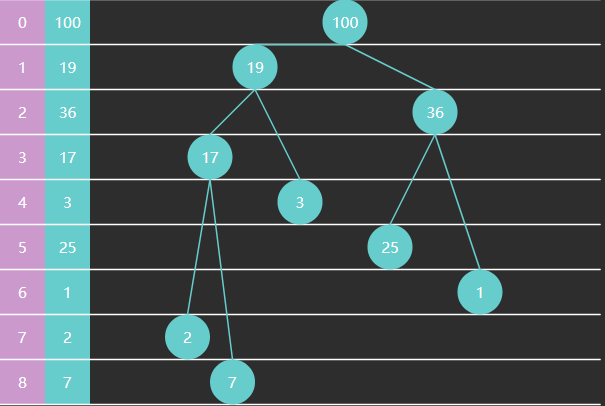
例3 - 大顶堆
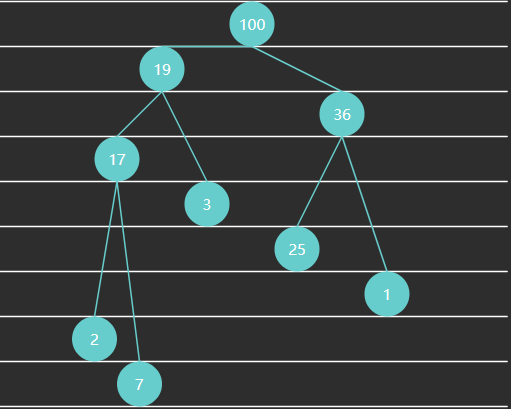
例4 - 小顶堆
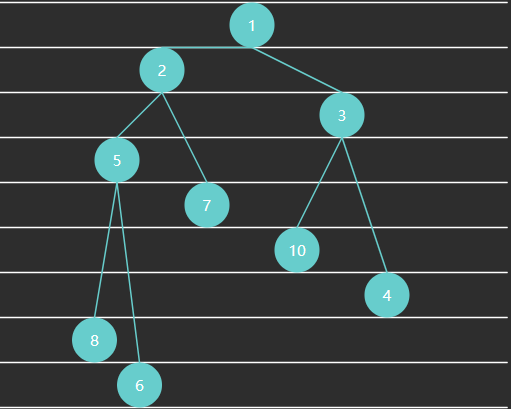
完全二叉树可以使用数组来表示
特征
- 如果从索引 0 开始存储节点数据
- 节点 的父节点为 ,当 时
- 节点 的左子节点为 ,右子节点为 ,当然它们得
- 如果从索引 1 开始存储节点数据
- 节点 的父节点为 ,当 时
- 节点 的左子节点为 ,右子节点为 ,同样得
代码
public class PriorityQueue4<E extends Priority> implements Queue<E> {
Priority[] array;
int size;
public PriorityQueue4(int capacity) {
array = new Priority[capacity];
}
@Override
public boolean offer(E offered) {
if (isFull()) {
return false;
}
int child = size++;
int parent = (child - 1) / 2;
while (child > 0 && offered.priority() > array[parent].priority()) {
array[child] = array[parent];
child = parent;
parent = (child - 1) / 2;
}
array[child] = offered;
return true;
}
private void swap(int i, int j) {
Priority t = array[i];
array[i] = array[j];
array[j] = t;
}
@Override
public E poll() {
if (isEmpty()) {
return null;
}
swap(0, size - 1);
size--;
Priority e = array[size];
array[size] = null;
shiftDown(0);
return (E) e;
}
void shiftDown(int parent) {
int left = 2 * parent + 1;
int right = left + 1;
int max = parent;
if (left < size && array[left].priority() > array[max].priority()) {
max = left;
}
if (right < size && array[right].priority() > array[max].priority()) {
max = right;
}
if (max != parent) {
swap(max, parent);
shiftDown(max);
}
}
@Override
public E peek() {
if (isEmpty()) {
return null;
}
return (E) array[0];
}
@Override
public boolean isEmpty() {
return size == 0;
}
@Override
public boolean isFull() {
return size == array.length;
}
}
3.3 阻塞队列
之前的队列在很多场景下都不能很好地工作,例如
- 大部分场景要求分离向队列放入(生产者)、从队列拿出(消费者)两个角色、它们得由不同的线程来担当,而之前的实现根本没有考虑线程安全问题
- 队列为空,那么在之前的实现里会返回 null,如果就是硬要拿到一个元素呢?只能不断循环尝试
- 队列为满,那么再之前的实现里会返回 false,如果就是硬要塞入一个元素呢?只能不断循环尝试
因此我们需�要解决的问题有
- 用锁保证线程安全
- 用条件变量让等待非空线程与等待不满线程进入等待状态,而不是不断循环尝试,让 CPU 空转
有同学对线程安全还没有足够的认识,下面举一个反例,两个线程都要执行入队操作(几乎在同一时刻)
public class TestThreadUnsafe {
private final String[] array = new String[10];
private int tail = 0;
public void offer(String e) {
array[tail] = e;
tail++;
}
@Override
public String toString() {
return Arrays.toString(array);
}
public static void main(String[] args) {
TestThreadUnsafe queue = new TestThreadUnsafe();
new Thread(()-> queue.offer("e1"), "t1").start();
new Thread(()-> queue.offer("e2"), "t2").start();
}
}
执行的时间序列如下,假设初始状态 tail = 0,在执行过程中由于 CPU 在两个线程之间切换,造成了指令交错
| 线程1 | 线程2 | 说明 |
|---|---|---|
| array[tail]=e1 | 线程1 向 tail 位置加入 e1 这个元素,但还没来得及执行 tail++ | |
| array[tail]=e2 | 线程2 向 tail 位置加入 e2 这个元素,覆盖掉了 e1 | |
| tail++ | tail 自增为1 | |
| tail++ | tail 自增为2 | |
| 最后状态 tail 为 2,数组为 [e2, null, null ...] |
糟糕的是,由于指令交错的顺序不同,得到的结果不止以上一种,宏观上造成混乱的效果
单锁实现
Java 中要防止代码段交错执行,需要使用锁,有两种选择
- synchronized 代码块,属于关键字级别提供锁保护,功能少
- ReentrantLock 类,功能丰富
以 ReentrantLock 为例
ReentrantLock lock = new ReentrantLock();
public void offer(String e) {
lock.lockInterruptibly();
try {
array[tail] = e;
tail++;
} finally {
lock.unlock();
}
}
只要两个线程执行上段代码时,锁对象是同一个,就能保证 try 块内的代码的执行不会出现指令交错现象,即执行顺序只可能是下面两种情况之一
| 线程1 | 线程2 | 说明 |
|---|---|---|
| lock.lockInterruptibly() | t1对锁对象上锁 | |
| array[tail]=e1 | ||
| lock.lockInterruptibly() | 即使 CPU 切换到线程2,但由于t1已经对该对象上锁,因此线程2卡在这儿进不去 | |
| tail++ | 切换回线程1 执行后续代码 | |
| lock.unlock() | 线程1 解锁 | |
| array[tail]=e2 | 线程2 此时才能获得锁,执行它的代码 | |
| tail++ |
- 另一种情况是线程2 先获得锁,线程1 被挡在外面
- 要明白保护的本质,本例中是保护的是 tail 位置读写的安全
事情还没有完,上面的例子是队列还没有放满的情况,考虑下面的代码(这回锁同时保护了 tail 和 size 的读写安全)
ReentrantLock lock = new ReentrantLock();
int size = 0;
public void offer(String e) {
lock.lockInterruptibly();
try {
if(isFull()) {
// 满了怎么办?
}
array[tail] = e;
tail++;
size++;
} finally {
lock.unlock();
}
}
private boolean isFull() {
return size == array.length;
}
之前是返回 false 表示添加失败,前面分析过想达到这么一种效果:
- 在队列满时,不是立刻返回,而是当前线程进入等待
- 什么时候队列不满了,再唤醒这个等待的线程,从上次的代码处继续向下运行
ReentrantLock 可以配合条件变量来实现,代码进化为
ReentrantLock lock = new ReentrantLock();
Condition tailWaits = lock.newCondition(); // 条件变量
int size = 0;
public void offer(String e) {
lock.lockInterruptibly();
try {
while (isFull()) {
tailWaits.await(); // 当队列满时, 当前线程进入 tailWaits 等待
}
array[tail] = e;
tail++;
size++;
} finally {
lock.unlock();
}
}
private boolean isFull() {
return size == array.length;
}
- 条件变量底层也是个队列,用来存储这些需要等待的线程,当队列满了,就会将 offer 线程加入条件队列,并暂时释放锁
- 将来我们的队列如果不满了(由 poll 线程那边得知)可以调用 tailWaits.signal() 来唤醒 tailWaits 中首个等待的线程,被唤醒的线程会再次抢到锁,从上次 await 处继续向下运行
思考为何要用 while 而不是 if,设队列容量是 3
| 操作前 | offer(4) | offer(5) | poll() | 操作后 |
|---|---|---|---|---|
| [1 2 3] | 队列满,进入tailWaits 等待 | [1 2 3] | ||
| [1 2 3] | 取走 1,队列不满,唤醒线程 | [2 3] | ||
| [2 3] | 抢先获得锁,发现不满,放入 5 | [2 3 5] | ||
| [2 3 5] | 从上次等待处直接向下执行 | [2 3 5 ?] |
关键点:
- 从 tailWaits 中唤醒的线程,会与新来的 offer 的线程争抢锁,谁能抢到是不一定的,如果后者先抢到,就会导致条件又发生变化
- 这种情况称之为虚假唤醒,唤醒后应该重新检查条件,看是不是得重新进入等待
最后的实现代码
/**
* 单锁实现
* @param <E> 元素类型
*/
public class BlockingQueue1<E> implements BlockingQueue<E> {
private final E[] array;
private int head = 0;
private int tail = 0;
private int size = 0; // 元素个数
@SuppressWarnings("all")
public BlockingQueue1(int capacity) {
array = (E[]) new Object[capacity];
}
ReentrantLock lock = new ReentrantLock();
Condition tailWaits = lock.newCondition();
Condition headWaits = lock.newCondition();
@Override
public void offer(E e) throws InterruptedException {
lock.lockInterruptibly();
try {
while (isFull()) {
tailWaits.await();
}
array[tail] = e;
if (++tail == array.length) {
tail = 0;
}
size++;
headWaits.signal();
} finally {
lock.unlock();
}
}
@Override
public void offer(E e, long timeout) throws InterruptedException {
lock.lockInterruptibly();
try {
long t = TimeUnit.MILLISECONDS.toNanos(timeout);
while (isFull()) {
if (t <= 0) {
return;
}
t = tailWaits.awaitNanos(t);
}
array[tail] = e;
if (++tail == array.length) {
tail = 0;
}
size++;
headWaits.signal();
} finally {
lock.unlock();
}
}
@Override
public E poll() throws InterruptedException {
lock.lockInterruptibly();
try {
while (isEmpty()) {
headWaits.await();
}
E e = array[head];
array[head] = null; // help GC
if (++head == array.length) {
head = 0;
}
size--;
tailWaits.signal();
return e;
} finally {
lock.unlock();
}
}
private boolean isEmpty() {
return size == 0;
}
private boolean isFull() {
return size == array.length;
}
}
- public void offer(E e, long timeout) throws InterruptedException 是带超时的版本,可以只等待一段时间,而不是永久等下去,类似的 poll 也可以做带超时的版本,这个留给大家了
注意
- JDK 中 BlockingQueue 接口的方法命名与我的示例有些差异
- 方法 offer(E e) 是非阻塞的实现,阻塞实现方法为 put(E e)
- 方法 poll() 是非阻塞的实现,阻塞实现方法为 take()
双锁实现
单锁的缺点在于:
- 生产和消费几乎是不冲突的,唯一冲突的是生产者和消费者它们有可能同时修改 size
- 冲突的主要是生产者之间:多个 offer 线程修改 tail
- 冲突的还有消费者之间:多个 poll 线程修改 head
如果希望进一步提高性能,可以用两把锁
- 一把锁保护 tail
- 另一把锁保护 head
ReentrantLock headLock = new ReentrantLock(); // 保护 head 的锁
Condition headWaits = headLock.newCondition(); // 队列空时,需要等待的线程集合
ReentrantLock tailLock = new ReentrantLock(); // 保护 tail 的锁
Condition tailWaits = tailLock.newCondition(); // 队列满时,需要等待的线程集合
先看看 offer 方法的初步实现
@Override
public void offer(E e) throws InterruptedException {
tailLock.lockInterruptibly();
try {
// 队列满等待
while (isFull()) {
tailWaits.await();
}
// 不满则入队
array[tail] = e;
if (++tail == array.length) {
tail = 0;
}
// 修改 size (有问题)
size++;
} finally {
tailLock.unlock();
}
}
上面代码的缺点是 size 并不受 tailLock 保护,tailLock 与 headLock 是两把不同的锁,并不能实现互斥的效果。因此,size 需要用下面的代码保证原子性
AtomicInteger size = new AtomicInteger(0); // 保护 size 的原子变量
size.getAndIncrement(); // 自增
size.getAndDecrement(); // 自减
代码修改为
@Override
public void offer(E e) throws InterruptedException {
tailLock.lockInterruptibly();
try {
// 队列满等待
while (isFull()) {
tailWaits.await();
}
// 不满则入队
array[tail] = e;
if (++tail == array.length) {
tail = 0;
}
// 修改 size
size.getAndIncrement();
} finally {
tailLock.unlock();
}
}
对称地,可以写出 poll 方法
@Override
public E poll() throws InterruptedException {
E e;
headLock.lockInterruptibly();
try {
// 队列空等待
while (isEmpty()) {
headWaits.await();
}
// 不空则出队
e = array[head];
if (++head == array.length) {
head = 0;
}
// 修改 size
size.getAndDecrement();
} finally {
headLock.unlock();
}
return e;
}
下面来看一个难题,就是如何通知 headWaits 和 tailWaits 中等待的线程,比如 poll 方法拿走一个元素,通知 tailWaits:我拿走一个,不满了噢,你们可以放了,因此代码改为
@Override
public E poll() throws InterruptedException {
E e;
headLock.lockInterruptibly();
try {
// 队列空等待
while (isEmpty()) {
headWaits.await();
}
// 不空则出队
e = array[head];
if (++head == array.length) {
head = 0;
}
// 修改 size
size.getAndDecrement();
// 通知 tailWaits 不满(有问题)
tailWaits.signal();
} finally {
headLock.unlock();
}
return e;
}
问题在于要使用这些条件变量的 await(), signal() 等方法需要先获得与之关联的锁,上面的代码若直接运行会出现以下错误
java.lang.IllegalMonitorStateException
那有同学说,加上锁不就行了吗,于是写出了下面的代码
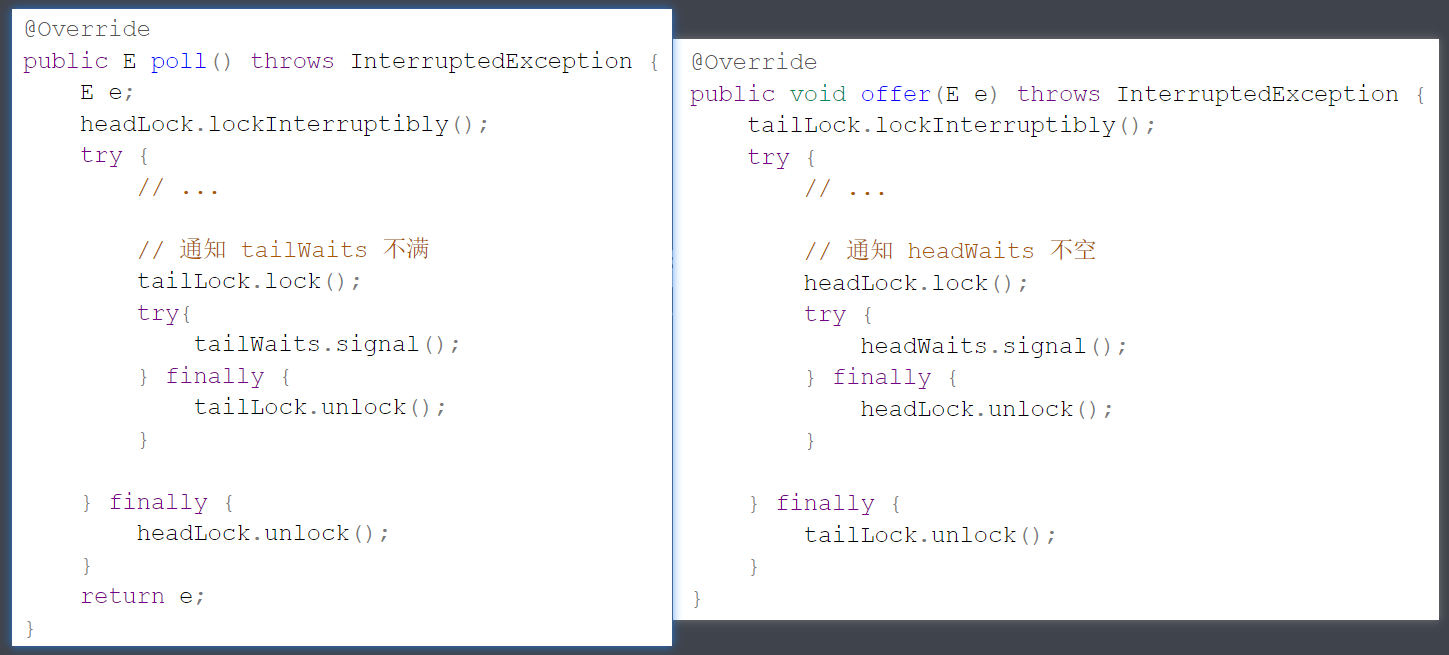
发现什么问题了?两把锁这么嵌套使用,非常容易出现死锁,如下所示
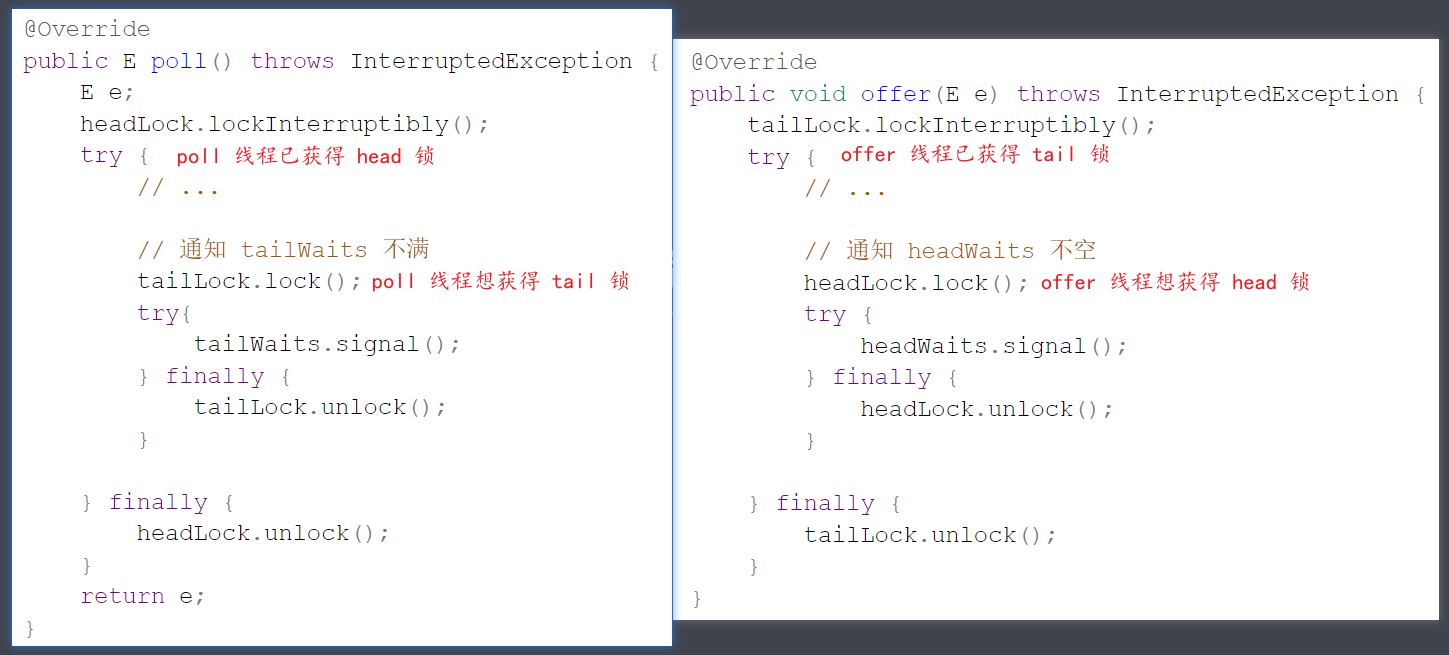
因此得避免嵌套,两段加锁的代码变成了下面平级的样子
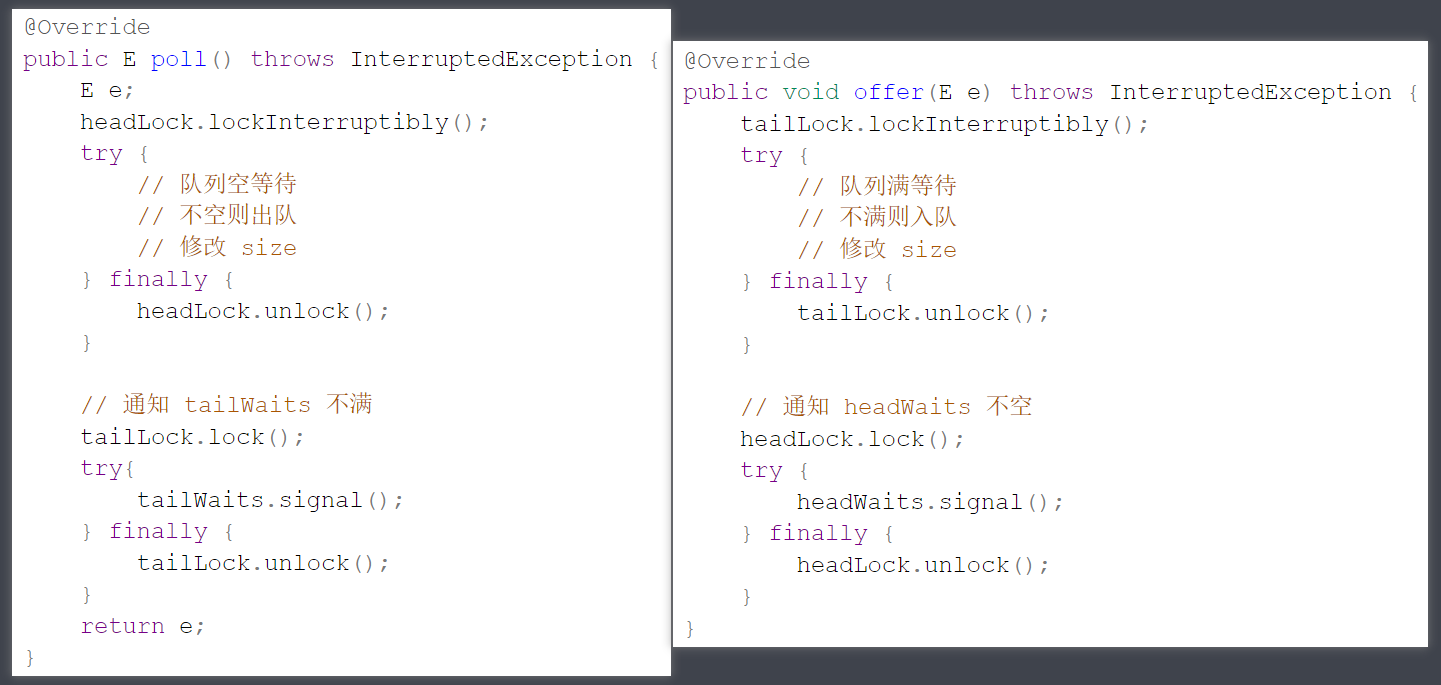
性能还可以进一步提升
-
代码调整后 offer 并没有同时获��取 tailLock 和 headLock 两把锁,因此两次加锁之间会有空隙,这个空隙内可能有其它的 offer 线程添加了更多的元素,那么这些线程都要执行 signal(),通知 poll 线程队列非空吗?
- 每次调用 signal() 都需要这些 offer 线程先获得 headLock 锁,成本较高,要想法减少 offer 线程获得 headLock 锁的次数
- 可以加一个条件:当 offer 增加前队列为空,即从 0 变化到不空,才由此 offer 线程来通知 headWaits,其它情况不归它管
-
队列从 0 变化到不空,会唤醒一个等待的 poll 线程,这个线程被唤醒后,肯定能拿到 headLock 锁,因此它具备了唤醒 headWaits 上其它 poll 线程的先决条件。如果检查出此时有其它 offer 线程新增了元素(不空,但不是从0变化而来),那么不妨由此 poll 线程来唤醒其它 poll 线程
这个技巧被称之为级联通知(cascading notifies),类似的原因
- 在 poll 时队列从满变化到不满,才由此 poll 线程来唤醒一个等待的 offer 线程,目的也是为了减少 poll 线程对 tailLock 上锁次数,剩下等待的 offer 线程由这个 offer 线程间接唤醒
最终的代码为
public class BlockingQueue2<E> implements BlockingQueue<E> {
private final E[] array;
private int head = 0;
private int tail = 0;
private final AtomicInteger size = new AtomicInteger(0);
ReentrantLock headLock = new ReentrantLock();
Condition headWaits = headLock.newCondition();
ReentrantLock tailLock = new ReentrantLock();
Condition tailWaits = tailLock.newCondition();
public BlockingQueue2(int capacity) {
this.array = (E[]) new Object[capacity];
}
@Override
public void offer(E e) throws InterruptedException {
int c;
tailLock.lockInterruptibly();
try {
while (isFull()) {
tailWaits.await();
}
array[tail] = e;
if (++tail == array.length) {
tail = 0;
}
c = size.getAndIncrement();
// a. 队列不满, 但不是从满->不满, 由此offer线程唤醒其它offer线程
if (c + 1 < array.length) {
tailWaits.signal();
}
} finally {
tailLock.unlock();
}
// b. 从0->不空, 由此offer线程唤醒等待的poll线程
if (c == 0) {
headLock.lock();
try {
headWaits.signal();
} finally {
headLock.unlock();
}
}
}
@Override
public E poll() throws InterruptedException {
E e;
int c;
headLock.lockInterruptibly();
try {
while (isEmpty()) {
headWaits.await();
}
e = array[head];
if (++head == array.length) {
head = 0;
}
c = size.getAndDecrement();
// b. 队列不空, 但不是从0变化到不空,由此poll线程通知其它poll线程
if (c > 1) {
headWaits.signal();
}
} finally {
headLock.unlock();
}
// a. 从满->不满, 由此poll线程唤醒等待的offer线程
if (c == array.length) {
tailLock.lock();
try {
tailWaits.signal();
} finally {
tailLock.unlock();
}
}
return e;
}
private boolean isEmpty() {
return size.get() == 0;
}
private boolean isFull() {
return size.get() == array.length;
}
}
双锁实现的非常精巧,据说作者 Doug Lea 花了一年的时间才完善了此段代码